College of Letters, Sciences, and Professional Studies (CLSPS)
Welcome to Montana Tech’s College of Letters, Sciences, and Professional Studies (CLSPS)!
CLSPS houses a wide array of programs spanning science, technology, and health. We’ve carefully designed each program with options to ensure our graduates gain market-ready skills for their chosen career path.
If you’re passionate about a career in the basic and applied sciences, you’ll find the perfect program here. Our customizable degrees can even incorporate studies in communications, business, social sciences, and humanities, allowing you to tailor your education to your unique interests.
The sheer breadth of educational opportunities within CLSPS is truly impressive, ready for you to pursue your passions. As you explore our programs and their various options, we’re confident you’ll find an opportunity that exceeds your expectations for your education, life, and career.
Dean's WelcomeProgram Brochures
Our Altitude Isn't Nearly as Breathtaking as Your Potential
Let's find out together. Schedule a visit or call us at 406-496-4791 to see if Montana Tech is the place you should be.

Biology at Montana Tech is a gateway to expansive possibilities in medicine, veterinary medicine, wildlife biology or graduate school.

This program offers a robust curriculum designed to strengthen your core business principles while integrating cutting-edge information technology and management of information systems. Students can specialize in Management Information Systems (MIS) and Accounting.

Students can choose from several areas of specialization, including biochemistry, environmental environmental chemistry, and a professional option.

With the growth in AI solutions, the market demand for data scientists is booming. Our programs, including a BS in Data Science and a Data Science minor, are crafted to integrate seamlessly with every science and engineering discipline, equipping you with essential skills for this exciting future.

Prepare to become either an Elementary or Secondary educator in Montana through the University of Montana-Western.

Gain a deep understanding of the science of human movement, preparing you for a range of professional opportunities in fields such as medicine, physical therapy, and other professional roles within healthcare and physical training.

The Department of Chemistry and Geochemistry offers a M.S. degree in Geochemistry.

Earn a well-rounded and multidisciplinary degree from a program that mixes science with the arts.
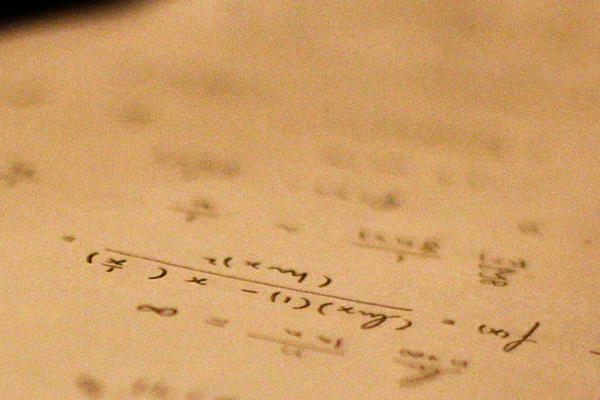
This degree prepares students for in-demand, high-paying careers. Focus on pure math, applied math, or statistics with this versatile degree.

Enter the exciting world of health care by becoming a registered nurse at Montana Tech.

The Writing Program aims to develop and improve the ability of all students to communicate effectively in academic and professional endeavors.

The pre-dentistry program at Montana Tech is designed to give students the science pre-requisites for acceptance into a dental school.

Focus your undergraduate studies on pre-law requirements.

A MPH degree trains professionals who tackle health issues that impact entire populations.

The pre-med program is a focused set of courses to qualify you for medical school.

Montana Tech has had a long history of placing students in pharmacy programs who were well prepared and are now practicing pharmacists.

Students interested in pursuing a career in physical therapy typically major in Exercise or Health Science or Biological Sciences.
Montana Tech’s faculty helps you tailor a plan of study to gain acceptance into physician's assistant programs.
This focus area is a gateway to a career in health or medicine like optometry, pharmacy, respiratory therapy, etc.

MTU has a strong Pre-Veterinary Medicine program, with many alumni who are now practicing veterinarians.
Faculty do more to determine the quality of your college experience than anyone. Here you will find dedicated and knowledgeable faculty, whose first priority is to give you an outstanding education that prepares you for success.
If you’re passionate about research, consider an undergraduate research project (URP) supported by grants from industry, the National Science Foundation, and the University. You can even earn a stipend!
If you’ve already earned your baccalaureate degree and are seeking entry to graduate school, start at Montana Tech. Two majors in the College of Letters, Sciences, and Professional Studies are available as master’s programs.
Accreditation
Accreditation varies by department. Please visit each program page for accreditation information.